Proposition 37

Triangles which are on the same base and in the same parallels equal one another.

Let ABC and DBC be triangles on the same base BC and in the same parallels AD and BC.

I.37

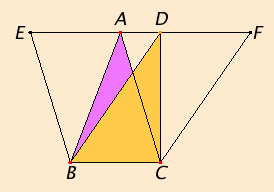
I say that the triangle ABC equals the triangle DBC.

I.Post.2
I.31

Produce AD in both directions to E and F. Draw BE through B parallel to CA, and draw CF through C parallel to BD.

I.35

Then each of the figures EBCA and DBCF is a parallelogram, and they are equal, for they are on the same base BC and in the same parallels BC and EF.

I.34

Moreover the triangle ABC is half of the parallelogram EBCA, for the diameter AB bisects it. And the triangle DBC is half of the parallelogram DBCF, for the diameter DC bisects it.

C.N

Therefore the triangle ABC equals the triangle DBC.

Therefore triangles which are on the same base and in the same parallels equal one another.

Q.E.D.

Guide

 In this proposition the triangles have the same base while in the next one the triangles have equal bases. Since the proofs are the same except that this depends on I.35 while the next depends on I.36, and the next is more general, there is no purpose to include this proposition.

The justification of the last conclusion is missing. From the statement that the doubles of two magnitudes are equal, we want to conclude that the magnitudes themselves are equal. Although Euclid included no such common notion, others inserted it later. See the commentary on Common Notions for a proof of this halving principle based on other properties of magnitudes.

Use of Proposition 37

This proposition is used in I.39, I.41, and VI.2.