Proposition 3

To circumscribe a triangle equiangular with a given triangle about a given circle.

Let ABC be the given circle, and DEF the given triangle.

It is required to circumscribe a triangle equiangular with the triangle DEF about the circle ABC.

java applet or image
III.1
I.23
III.16,Cor

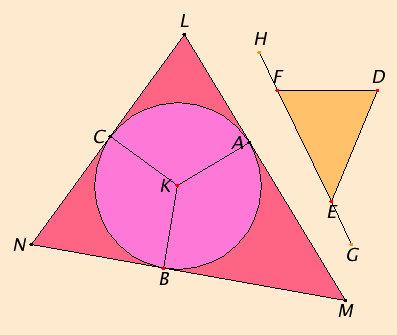
Produce EF in both directions to the points G and H. Take the center K of the circle ABC, and draw a radius KB at random. On the straight line KB and at the point K on it, construct the angle BKA equal to the angle DEG, and the angle BKC equal to the angle DFH. Through the points A, B, and C draw LAM, MBN, and NCL touching the circle ABC.

III.18

Now, since LM, MN, and NL touch the circle ABC at the points A, B, and C, and KA, KB, and KC have been joined from the center K to the points A, B, and C, therefore the angles at the points A, B, and C are right.

And, since the four angles of the quadrilateral AMBK equal four right angles, inasmuch as AMBK is in fact divisible into two triangles, and the angles KAM and KBM are right, therefore the sum of the remaining angles AKB and AMB equals two right angles.

I.13

But the sum of the angles DEG and DEF also equals two right angles, therefore the sum of the angles AKB and AMB equals the sum of the angles DEG and DEF, of which the angle AKB equals the angle DEG, therefore the remaining angle AMB equals the remaining angle DEF.

I.32

Similarly it can be proved that the angle LNB also equals the angle DFE, therefore the remaining angle MLN equals the angle EDF.

IV.Def.4

Therefore the triangle LMN is equiangular with the triangle DEF, and it has been circumscribed about the circle ABC.

Therefore a triangle equiangular with the given triangle has been circumscribed about a given circle.

Q.E.F.

Guide

This proposition is not used elsewhere in the Elements, but is included as a mate to the previous proposition in which a triangle is inscribed inside rather than circumscribed outside a given circle.